Answer:
a) 0.3889
b) 0.5
c) 0.8333
d) The mean is 250 and the standard deviation is 51.96.
Explanation:
An uniform probability is a case of probability in which each outcome is equally as likely.
For this situation, we have a lower limit of the distribution that we call a and an upper limit that we call b.
The probability of finding a value of X higher than x is:

The probability of finding a value of X between c and d is:
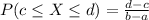
The mean and the standard deviation are, respectively:
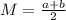

A random variable follows the continuous uniform distribution between 160 and 340.
This means that

a)

b)

c)

d)
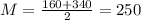

The mean is 250 and the standard deviation is 51.96.