Answer:
8, 7.92, 2.81
Explanation:
For each Social Security recipient, there are only two possible outcomes. Either they are too young to vote, or they are not. The probability of a Social Security recipient is independent of any other Social Security recipient. So we use the binomial probability distribution to solve this question.
Binomial probability distribution
Probability of exactly x sucesses on n repeated trials, with p probability.
The expected value of the binomial distribution is:
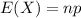
The variance of the binomial distribution is:
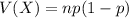
The standard deviation of the binomial distribution is:
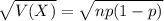
In this problem, we have that:
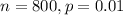
So
Mean:
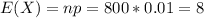
The variance of the binomial distribution is:
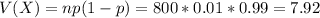
The standard deviation of the binomial distribution is:
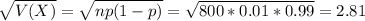
Formatted answer: 8, 7.92, 2.81