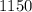Answer:
The estimated total rabbit population would be
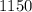
Step-by-step explanation:
Given -
Total number of rabbit marked (m)

Total number of marked rabbit recaptured(n)
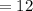
Total number of initially marked rabbit (M)
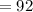
Let the total rabbit population be "N"
Now as we know that
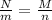
On rearranging, we get the following equation -

Substituting the given values in above equation we get -
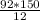
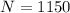
The estimated total rabbit population would be