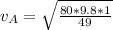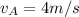Question

Block B is attached to a massless string of length L = 1 m and is free to rotate as a pendulum. The speed of block A after the collision is half its speed before the collision. Block B was at rest before the collision. The mass of block A is 7 kg and the mass of block B is 2 kg. What is the minimum initial speed (in m/s) that block A must have for block B to swing through a complete vertical circle?

Answer 1

Complete Question

The diagram for this question is shown on the first uploaded image

Answer:

The minimum velocity of A is
`v_A= 4m/s`

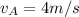
Step-by-step explanation:

From the question we are told that

The length of the string is
`L = 1m`

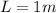
The initial speed of block A is
`u_A`

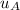
The final speed of block A is
`v_A = (1)/(2)u_A`

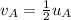
The initial speed of block B is
`u_B = 0`

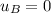
The mass of block A is
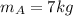`m_A = 7kg` gh

The mass of block B is
`m_B = 2 kg`

According to the principle of conservation of momentum

`m_A u_A + m_B u_B = m_Bv_B + m_A (u_A)/(2)`

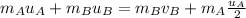
Since block B at initial is at rest

`m_A u_A = m_Bv_B + m_A (u_A)/(2)`

`m_A u_A - m_A (u_A)/(2) = m_Bv_B`

`m_A (u_A)/(2) = m_Bv_B`

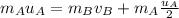
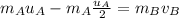
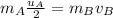
making
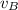`v_B` the subject of the formula

`v_B =m_A (u_A)/(2 m_B)`

Substituting values

`v_B =(7 u_A)/(4)`

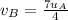
This
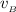`v__B` is the velocity at bottom of the vertical circle just at the collision with mass A

Assuming that block B is swing through the vertical circle(shown on the second uploaded image ) with an angular velocity of
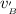`v__B'` at the top of the vertical circle

The angular centripetal acceleration would be mathematically represented

`a= (v^2_(B)')/(L)`

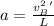
Note that this acceleration would be toward the center of the circle

Now the forces acting at the top of the circle can be represented mathematically as

`T + mg = m (v^2_(B)')/(L)`

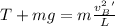
Where T is the tension on the string

According to the law of energy conservation

The energy at bottom of the vertical circle = The energy at the top of

the vertical circle

This can be mathematically represented as

`(1)/(2) m(v_B)^2 = (1)/(2) mv^2_B' + mg 2L`

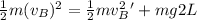
From above

`(T + mg) L = m v^2_(B)'`

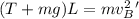
Substitute this into above equation

`(1)/(2) m((7 v_A)/(4) )^2 = (1)/(2) (T + mg) L + mg 2L`

`(49 mv_A^2)/(16) = (1)/(2) (T + mg) L + mg 2L`

`(49 mv_A^2)/(16) = T + 5mgL`

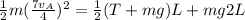
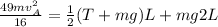
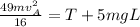
The value of velocity of block A needed to cause B be to swing through a complete vertical circle is would be minimum when tension on the string due to the weight of B is zero

This is mathematically represented as

`(49 mv_A^2)/(16) = 5mgL`

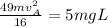
making
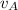`v_A` the subject

`v_A = \sqrt{(80mgL)/(49m) }`

substituting values

`v_A = \sqrt{(80* 9.8 *1)/(49) }`

`v_A= 4m/s`