Answer:
For this case we have the following info related to the time to prepare a return
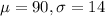
And we select a sample size =49>30 and we are interested in determine the standard deviation for the sample mean. From the central limit theorem we know that the distribution for the sample mean
 is given by:
is given by:
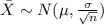
And the standard deviation would be:
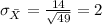
And the best answer would be
b. 2 minutes
Explanation:
Previous concepts
Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".
The central limit theorem states that "if we have a population with mean μ and standard deviation σ and take sufficiently large random samples from the population with replacement, then the distribution of the sample means will be approximately normally distributed. This will hold true regardless of whether the source population is normal or skewed, provided the sample size is sufficiently large".
Solution to the problem
For this case we have the following info related to the time to prepare a return
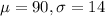
And we select a sample size =49>30 and we are interested in determine the standard deviation for the sample mean. From the central limit theorem we know that the distribution for the sample mean
 is given by:
is given by:
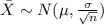
And the standard deviation would be:
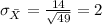
And the best answer would be
b. 2 minutes