Question

An astronaut on the moon throws a baseball upward. The astronaut is 6 ft 6 in tall, and the initial velocity of the ball is 40 ft per sec. The height s of the ball in feet is

given by the equation s = -2.7t^2 + 40t + 6.5, where t is the number of seconds after the ball was thrown. Complete parts a and b.

a. After how many seconds is the ball 14 ft above the moon's surface?

After ____ seconds the ball will be 14 ft above the moon's surface.

(Round to the nearest hundredth as needed. Use a comma to separate answers as needed.)

Answer 1

Answer: 0.19s and 14.6s

Explanation:

The distance of the ball at any time is given by the function s(t). For now, you can ignore all the details about the height of the man and the intial velocity of the ball. We only care about what time 't' will the ball be 14 feet off the ground. In math terms, they are asking when:

`s(t)=14`

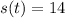
Where:

`s(t)=-2.7t^2+40t+6.5=14`

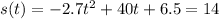
Subtract 14 on both sides:

`s(t)=-2.7t^2+40t-7.5=0`

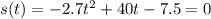
Use the quadratic formula to solve for the variable 't'. This will give the possible time values where the ball will be 14 feet off the ground:

`t=-b+√(b^2-4ac) /2a`

`t=-b-√(b^2-4ac) /2a`

`a=-2.7`

`b=40`

`c=-7.5`

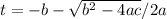
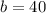
Therefore:

`t=(-40)+√(40^2-4(-2.7)(-7.5)) /2(-2.7)=0.19s`

`t=-40-√(40^2-4(-2.7)(-7.5)) /2(-2.7)=14.6s`

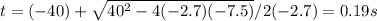
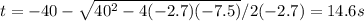
This is saying the ball almost immediately hits 14 feet given the intial velocity, then it reaches its peak and falls back down to 14 feet again, where it will eventually hit the ground again