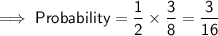2 Answers

Mike Bartlett · Answer 1 · 2023-12-11T08:38:58+0000

Answer:

A

Explanation:

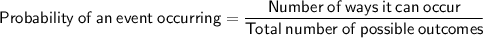
$\sf Probability\:of\:an\:event\:occurring = (Number\:of\:ways\:it\:can\:occur)/(Total\:number\:of\:possible\:outcomes)$

The spinner is evenly divided and numbered from 1 through 8.

$\sf \implies Probability\:of\:landing\:on\:any\:number= \frac18$

There are 4 even numbers (2, 4, 6 and 8)

$\sf \implies Probability\:of\:landing\:on\:an\:even\:number= \frac48=\frac12$

There are 3 numbers less than 4 (1, 2 and 3)

$\sf \implies Probability\:of\:landing\:on\:a\:number\:less\:than\:4=\frac38$

Therefore, the probability of landing of an even number and then on a number of less than 4 is:

$\sf \implies Probability=\frac12 * \frac38=(3)/(16)$

Please log in or register to add a comment.