Question

If m is the midpoint of segment JK, JM equals 7x minus 32, and MK equals 89 minus 4x, what is MK?

Answer 1

Explanation:

Imagine this segment, given that m is the midpoint of segment JK...

J________M________K

JM = 7x-32 MK = 89 - 4x (given)

If m is the midpoint, then JM and MK are the same lengths (equal to each other). We can use that to first find what x is.

`7x -32 = 89 - 4x\\`

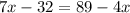
Steps to find x (we have to get x by itself):

`7x - 32 + 4x = 89 - 4x +4x`

`11x -32 = 89`

`11x -32 +32 = 89 +32`

`11x = 121`

`(11x)/(11) = (121)/(11)`

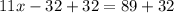
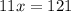
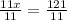
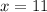`x=11` (we haven't found the answer yet! we only found x)

Now that we found x, we can find segment MK. Now we know that:

`MK = 89 - 4x`

`x= 11`

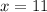
Let's plug x into the equation:

`MK = 89 - 4(11)\\MK = 89- (44)\\MK = 45`

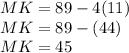
Answer:
`MK= 45`