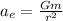Question

Tidal forces are gravitational forces exerted on different parts of a body by a second body. Their effects are particularly visible on the earth's surface in the form of tides. To understand the origin of tidal forces, consider the earth-moon system to consist of two spherical bodies, each with a spherical mass distribution. Let re be the radius of the earth, m be the mass of the moon, and G be the gravitational constant.

Let r denote the distance between the center of the earth and the center of the moon. What is the magnitude of the acceleration the gravitational pull of the moon?

Answer 1

`a_e = (Gm)/(r^2)`

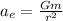
Step-by-step explanation:

We assume that:

M to represent the mass of the earth

m to equally represent the mass of the moon

r should be the distance between the center of the earth to the center of the moon.

Then;

the expression for the gravitational force can be written as:

`F = (GMm)/(r^2)`

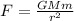
Where
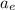`a_e` is the acceleration produced by the earth; then:

`F =M *a_e`

Then:

`M*a_e = (GMm)/(r^2)`

`a_e = (GMm)/(Mr^2)`

`a_e = (Gm)/(r^2)`

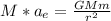
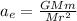
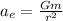
Therefore, the magnitude of the acceleration of the earth due to the gravitational pull of the moon
`a_e = (Gm)/(r^2)`

Answer 2

The magnitude of the acceleration of earth due to the gravitational pull of earth is a = Gm/r^2

Where r = the center to center distance between the earth and the moon,

m = mass of the moon, and,

G is the gravity constant.

Step-by-step explanation:

Detailed explanation and calculation is shown in the image below