Answer:
Option A)
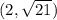
Explanation:
The following information is missing in the question:
A.
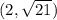
B.
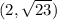
C. (2, 1)
D. (2, 3)
We are given the following in the question:
A circle centered at origin and radius 5 units.
We have to find the equation of a point that lies on the circle.
Let (x,y) lie on the circle.
Distance formula:

Putting
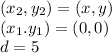
We get,

is the required equation of point on the circle centered at the origin with a radius of 5 units.
The point
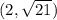 satisfies the given equation.
satisfies the given equation.
Verification:

Thus,
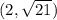 lies on the circle centered at the origin with a radius of 5 units.
lies on the circle centered at the origin with a radius of 5 units.