Question

The Wall Street Journal recently published an article indicating differences in perception of sexual harassment on the job between men and women. The article claimed that women perceived the problem to be much more prevalent than did men. One question asked of both men and women was: "Do you think sexual harassment is a major problem in the American workplace?" 24% of the men compared to 62% of the women responded "Yes". Suppose that 150 women and 200 men were interviewed.

a) What are the null and alternative hypotheses that The Wall Street Journal should test in order to show that its claim is true?
b) For a 0.01 level of significance, what is the critical value for the rejection region?
c) What is the value of the test statistic? (hint: you need to find pbar)
d) What is the p-value of the test?
e) What conclusion should be reached?

Answer 1

(a) H₀: P₂ - P₁ = 0 vs. Hₐ: P₂ - P₁ > 0.

(b) The critical value for the rejection region is 2.33.

(c) The calculated z-statistic value is, z = 7.17.

(d) The p-value of the test is 0.

(e) The proportion of women who view sexual harassment on the job is more than that for men.

Explanation:

Here we need to test whether the proportion of women who view sexual harassment on the job is more than that for men.

(a)

Our hypothesis will be:

H₀: The difference between the proportions of men and women who view sexual harassment on the job as a problem is same, i.e. P₂ - P₁ = 0

Hₐ: The difference between the proportions of men and women who view sexual harassment on the job as a problem is more than 0, i.e. P₂ - P₁ > 0.

(b)

The significance level of the test is:

α = 0.01

The rejection region is defined as:

If test statistic value, z
`_(t)` > z₀.₀₁ then then null hypothesis will be rejected.

Compute the critical value of the test as follows:

`z_(\alpha)=z_(0.01)=2.33`

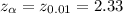
*Use z-table.

Thus, the critical value for the rejection region is 2.33.

(c)

The z-statistic for difference of proportions is,

`z=\frac{\hat p_(2)-\hat p_(1)}{\sqrt{P(1-P)* ((1)/(n_(2))+(1)/(n_(1)))}}`

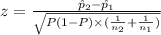
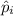`\hat p_(i)` = ith sample proportion,

P = population proportion

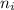`n_(i)` = ith sample size.

The given information is:

`n_(1)=200\\n_(2)=150\\\hat p_(1)=0.24\\\hat p_(2)=0.62`

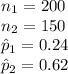
Since, there is no data about the population proportion the unbiased estimate of P is given by,

`P=(n_(1)\hat p_(1)+n_(2)\hat p_(2))/(n_(1)+n_(2))=(200* 0.24+150* 0.62)/(200+150)=0.4029`

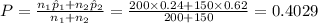
Using the given data we compute the z-statistic as:

`z=\frac{\hat p_(2)-\hat p_(1)}{\sqrt{P(1-P)* ((1)/(n_(2))+(1)/(n_(1)))}}`

`=\frac{0.62-0.24}{\sqrt{0.4029(1-0.4029)* ((1)/(150)+(1)/(200))}}`

`=7.17`

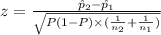
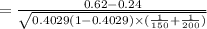
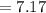
Thus, the calculated z-statistic value is, z = 7.17.

(d)

Compute the p-value of the test as follows:

`p-value=P(Z>z_(t))`

`=P(Z>7.17)\\=1-P(Z<7.17)\\=1 -(\approx1)\\=0`

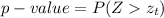
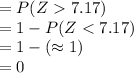
Thus, the p-value of the test is 0.

(e)

As stated in part (b), if z₀.₀₁ > z
`_(t)` then then null hypothesis will be rejected.

z
`_(t)` = 7.17 > z₀.₀₁ = 2.33

Thus, the null hypothesis will be rejected at 1% level of significance.

Conclusion:

The proportion of women who view sexual harassment on the job is more than that for men.