Answer:
1) The solubility product of the lead(II) chloride is
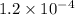 .
.
2) The solubility of the aluminium hydroxide is
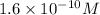 .
.
3)The given statement is false.
Step-by-step explanation:
1)
Solubility of lead chloride =

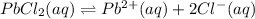
S 2S
The solubility product of the lead(II) chloride =

![K_(sp)=[Pb^(2+)][Cl^-]^2](https://img.qammunity.org/2021/formulas/chemistry/college/bnzapmsqdui2w7g2cdfebjt14frtn40x1e.png)
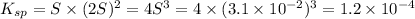
The solubility product of the lead(II) chloride is
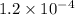 .
.
2)
Concentration of aluminium nitrate = 0.000010 M
Concentration of aluminum ion =
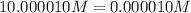
Solubility of aluminium hydroxide in aluminum nitrate solution =

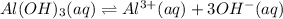
S 3S
The solubility product of the aluminium nitrate =
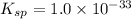
![K_(sp)=[Al^(3+)][OH^-]^3](https://img.qammunity.org/2021/formulas/chemistry/high-school/nu322j7dfbog1yncqihy91vlyg66nz3e1x.png)
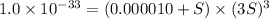
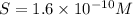
The solubility of the aluminium hydroxide is
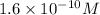 .
.
3.
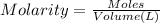
Mass of NaCl= 3.5 mg = 0.0035 g
1 mg = 0.001 g
Moles of NaCl =

Volume of the solution = 0.250 L
![[NaCl]=(6.0* 10^(-5) mol)/(0.250 L)=0.00024 M](https://img.qammunity.org/2021/formulas/chemistry/high-school/pmkbr2bpab7343itoas4pya5n9i9z3h5aa.png)
1 mole of NaCl gives 1 mole of sodium ion and 1 mole of chloride ions.
![[Cl^-]=[NaCl]=0.00024 M](https://img.qammunity.org/2021/formulas/chemistry/high-school/u1wl6o32qlqv5skoyyipsw743q8wgsi4ao.png)
Moles of lead (II) nitrate = n
Volume of the solution = 0.250 L
Molarity lead(II) nitrate = 0.12 M
![n=0.12 M]* 0.250 L=0.030 mol](https://img.qammunity.org/2021/formulas/chemistry/high-school/yomymr3q2axt7pobcm838iaq5tywx6ana7.png)
1 mole of lead nitrate gives 1 mole of lead (II) ion and 2 moles of nitrate ions.
![[Pb^(2+)]=[Pb(NO_2)_3]=0.030 M](https://img.qammunity.org/2021/formulas/chemistry/high-school/2u6nxdlrtrmhcphiaw9en63w37s4s3m987.png)
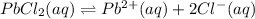
Solubility of lead(II) chloride =
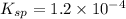
Ionic product of the lead chloride in solution :
![Q_i=[Pb^(2+)][Cl^-]^2=0.030 M* (0.00024 M)^2=1.7* 10^(-9)](https://img.qammunity.org/2021/formulas/chemistry/high-school/3yep63x2gqfhcjznty1q4xj8zktn4ip1rx.png)
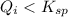 ( no precipitation)
( no precipitation)
The given statement is false.