Answer:
68
Explanation:
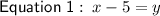

Substitute Equation 1 into Equation 2:
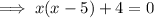
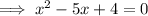
Factorize:
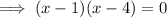
Therefore:
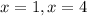
Substitute found values of
 into Equation 1 and solve for
into Equation 1 and solve for
 :
:
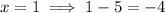

Substitute found values into
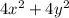 and solve:
and solve:
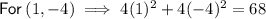
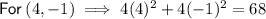
Note: As
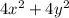 , the values of x and y can be interchanged, so no need to input both sets of ordered pairs into the equation to solve.
, the values of x and y can be interchanged, so no need to input both sets of ordered pairs into the equation to solve.