Question

3) You need to paint shutters on a window that is on the second floor of your house. You have a 20 foot ladder that you will use. There is a warning on the ladder that states the angle formed by the ladder and the ground must not be less than 70 degrees, or the ladder may slip and cause serious injury or death. You planned on placing the bottom of the ladder 3 feet from the base of the house. Will the angle formed between the ground and the ladder be safe? What is the furthest possible distance the ladder can be placed to maintain a safe angle? Sketch:​

Answer 1

See below ↓↓

Explanation:

• Height of ladder = 20 feet
• Distance of base of ladder from house = 3 feet
• Angle must not be < 70°

Taking the cos ratio of the angle

• Let the angle formed be α
• cos α = adjacent side / hypotenuse
• cos α = 3 / 20 = 0.15
• α = cos⁻¹ (0.15)
• α = 81.37°

⇒ The angle formed is safe

Finding furthest possible distance

• Take α to be 70°
• cos 70° = 0.34 = x/20
• ⇒ x = 20 x 0.34 = 6.8 feet

Answer 2

Yes, the angle formed between the ground and the ladder is safe

6.84 ft (nearest hundredth)

Explanation:

We can use the cos trig ratio to determine the angle made between the 20ft ladder and 3ft from the base of the house (see first attached image for sketch).

`\sf \cos(\theta)=(A)/(H)`

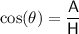
where:

• 
  `\theta` is the angle
• A is the side adjacent to the angle
• H is the hypotenuse

Given:

• A = 3 ft
• H = 20 ft

`\sf \implies \cos(\theta)=(3)/(20)`

`\sf \implies \theta=\cos^(-1)\left((3)/(20)\right)`

`\sf \implies \theta=81.37307344..^(\circ)`

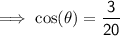
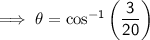
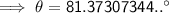
Therefore, as the ladder is forming an 81.37° angle and 81.37...° > 70° the angle formed between the ground and the ladder is safe.

To find the furthest possible distance the ladder can be placed, set
`\theta` to 70° (max safe angle) and the hypotenuse (ladder length) to 20, then solve for A (see second attache image for sketch):

`\sf \implies \cos(70^(\circ))=(A)/(20)`

`\sf \implies A=20\cos(70^(\circ))`

`\sf \implies A=6.840402867...\: \sf ft`

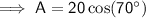
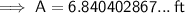
So the furthest possible distance the ladder can be placed to maintain a safe angle is 6.84 ft (nearest hundredth).