Question

What is the wavelength in nm of a light whose first order bright band forms a diffraction angle of 30 degrees, and the diffraction grating has 700 lines per mm?

Answer 1

Answer: 714.285 nm

Step-by-step explanation:

The diffraction angles
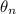`\theta_(n)` when we have a slit divided into
`n` parts are obtained by the following equation:

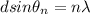`d sin\theta_(n)=n \lambda` (1)

Where:

`d` is the width of the slit

`\lambda` is the wavelength of the light

`n` is an integer different from zero

Now, the first-order diffraction angle is given when
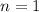`n=1`, hence equation (1) is rewritten as:

`dsin\theta_(1)=\lambda` (2)

We know:

`\theta_(1)=30\°`

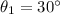
In addition we are told the diffraction grating has 700 lines per mm, this means:

`d=(1mm)/(700)`

Solving (2) with the known values we will find
`\lambda`:

`\lambda=((1mm)/(700))sin(30\°)` (3)

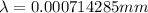`\lambda=0.000714285 mm` (4)

Knowing
`1mm=10^(6)nm`:

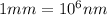`\lambda=714.285 nm` This is the wavelength of the light