Question

Two vertical parallel plates are spaced 0.01 ft apart. If the pressure decreases at a rate of 60 psf/ft in the vertical z-direction in the fluid between the plates, what is the maximum fluid velocity in the z-direction? The fluid has a viscosity of 10-3 lbf-s/ft2 and a specific gravity of 0.80.

Answer 1

umax = 0.1259ft/s

Step-by-step explanation:

Given:

•Distance between plates, B = 0.01ft

•Pressure difference decrease,
`(dp)/(dz)=60ps/ft`

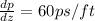
•Fluid viscosity, u = 10^-³lbf-s/ft²

Specific gravity, S = 0.80

Max velocity in the z-direction will be:

`u_max= [(B^2y)/(8u)](dh)/(ds)`

`But h = (P)/(y)+z`

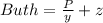
Substituting for h in the first equation, we have:

`(d)/(dz)[(p)/(y)+z]`

`(dh)/(dz)=(1)/(y)(dp)/(ds)+(dz)/(dz)`

`= (1)/(0.8*62.4)(-60)+1`

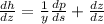
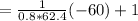
= -0.20192

Substituting dh/dz value in the first equation (umax), we have:

`umax = (0.01^2(0.8*62.4))/(8*10^-^3)(-0.20192)`

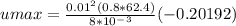
umax = 0.1259ft/s