Given:
In the circle P, ABCD is inscribed quadrilateral.
And, ∠DAB = 110°, ∠ABC = 72°
To find the value of ∠ADC.
Theorem:
A quadrilateral can be inscribed in a circle if and only if the opposite angles are supplementary [ sum of the opposite angles will be 180°]
According to the theorem,
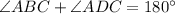

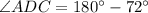
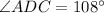
Hence,
The value of ∠ADC is 108°.
Hence, Option b is the correct answer.