Question

According to a recent​ census, 14.6​% of all housing units in a certain country are vacant. A county supervisor wonders if her county is different from this. She randomly selects 865 housing units in her county and finds that 159 of the housing units are vacant.

Name the model and check appropriate conditions for a hypothesis test. What kind of test is this?

A. One-proportion z-test
B. Two-proportion t-test
C. Proportional t-test
D. Difference in proportions test

Answer 1

We need to conduct a hypothesis in order to test the claim that the true proportion is equal to 14.6% or not. So we need to use a one proportion z test and the system of hypothesis are:

Null hypothesis:
`p=0.146`

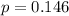
Alternative hypothesis:
`p \\eq 0.146`

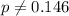
A. One-proportion z-test

When we conduct a proportion test we need to use the z statisitc, and the is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

And the conditions required are:

1) The data comes from a random sampling

2) Independence condition between observations

3) np>10 and n(1-p)>10

4) The sample size is 10 times lower than the population size.

Explanation:

Data given and notation

n=865 represent the random sample taken

X=159 represent the housing units that are vacant

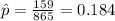`\hat p=(159)/(865)=0.184` estimated proportion of vacant units

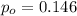`p_o=0.146` is the value that we want to test

`\alpha` represent the significance level

z would represent the statistic (variable of interest)

`p_v` represent the p value (variable of interest)

Solution to the problem

We need to conduct a hypothesis in order to test the claim that the true proportion is equal to 14.6% or not. So we need to use a one proportion z test and the system of hypothesis are:

Null hypothesis:
`p=0.146`

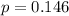
Alternative hypothesis:
`p \\eq 0.146`

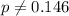
A. One-proportion z-test

When we conduct a proportion test we need to use the z statisitc, and the is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

And the conditions required are:

1) The data comes from a random sampling

2) Independence condition between observations

3) np>10 and n(1-p)>10

4) The sample size is 10 times lower than the population size.