Answer: The confidence interval would be (0.221, 0.279).
Explanation:
Since we have given that
n = 840
p = 0.25
q = 1 - p =

Let 95% level confidence, z = 1.96
Margin of error is given by :
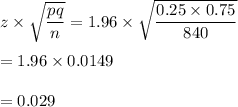
So, confidence interval would be

Hence, the confidence interval would be (0.221, 0.279).