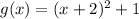Given:
The given parent function is
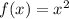
We need to determine the equation of the new translated (shifted) function g(x).
Vertical stretch:
The general rule to shift the graph f(x), to shift c units upward is
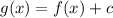
From the graph, it is obvious that the graph f(x) is shifted 1 unit upwards.
Thus, applying the above rule, we get;
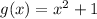
Horizontal stretch:
The general rule to shift the graph f(x) to shift c units to the left is
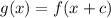
From, the graph, it is obvious that the graph f(x) is shifted 2 units to the left.
Thus, applying the above rule, we have;
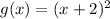
Equation of the new function g(x):
From the figure, it is obvious that the graph g(x) is shifted 1 unit upwards and 2 units to the left.
Thus, we have;
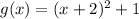
Therefore, the equation of the new function g(x) is