Question

A bucket of mass m is attached to a rope that is wound around the outside of a solid sphere (I = 2/5 M^2) of radius R. When the bucket is allowed to fall from rest, it falls with an acceleration of a down. What is the mass of the sphere in terms of m, R, a, and g?

Answer 1

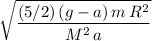`\displaystyle \sqrt{((5/2)\, (g - a)\, m\, R^(2))/(M^(2)\, a)}`, assuming that the tension in the rope is the only tangential force on the sphere (
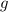`g` denote the gravitational acceleration.)

Step-by-step explanation:

The forces on the bucket are:

• Weight of the bucket:
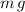  `m\, g` (downward.)
• Tension in the rope (upward.)

Since the weight of the bucket and the tension from the rope are in opposite directions, the magnitude of the net force would be:

`\begin{aligned} \|\text{Net Force}\| =\; & \|\text{Weight}\| - \|\text{Tension}\| \end{aligned}`.

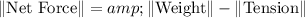The upward tension in the rope prevents the bucket from accelerating at
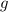`g` (free fall.) Rather, the bucket is accelerating at an acceleration of only
`a`. The net force on the bucket would be thus
`m\, a`.

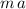Rearrange the equation for the net force on the bucket to find the magnitude of the tension in the rope would be:

`\begin{aligned} & \|\text{Tension}\| \\ =\; & \|\text{Weight}\| - \|\text{Net Force}\| \\ =\; & m\, g - m\, a \\ =\; & (g - a)\, m\end{aligned}`.

At a distance of
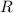`R` from the center of the sphere, the tension in the rope
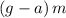`(g - a)\, m` would exert a torque of
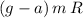`(g - a)\, m\, R` on the sphere. If this tension is the only tangential force on this sphere, the net torque on the sphere would be
`(g - a)\, m\, R\!`.

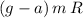Let
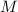`M` denote the mass of this sphere. The moment of inertia of this filled sphere would be
`I = (2/5)\, M^(2)`.

Therefore, the magnitude of the angular acceleration of this sphere would be:

`\begin{aligned}& \|\text{Angular Acceleration}\| \\ =\; & \frac{\|\text{Net Torque}\|}{(\text{Moment of Inertia})} \\ =\; & ((g - a)\, m\, R)/((2/5)\, M^(2)) \end{aligned}`.

The bucket is accelerating at a magnutide of
`a` downwards. The rope around the sphere need to unroll at an acceleration of the same magnitude,
`a\!`. The tangential acceleration of the sphere at the surface would also need to be
`\! a`.

Since the surface of the sphere is at a distance of
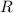`R` from the center, the angular acceleration of this sphere would be
`(a / R)`.

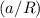Hence the equation:

`\begin{aligned}& ((g - a)\, m\, R^(2))/((2/5)\, M^(2)) = \|\text{Angular Acceleration}\| = (a)/(R) \end{aligned}`.

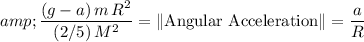Solve this equation for
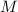`M`, the mass of this sphere:

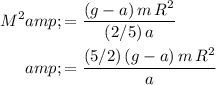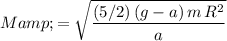`\begin{aligned}& ((g - a)\, m\, R^(2))/((2/5)\, M^(2)) = (a)/(R) \end{aligned}`.

`\begin{aligned}M^(2) &= ((g - a)\, m\, R^(2))/((2/5)\, a) \\ &= ((5/2)\, (g - a)\, m\, R^(2))/(a)\end{aligned}`.

`\begin{aligned}M&= \sqrt{((5/2)\, (g - a)\, m\, R^(2))/(a)}\end{aligned}`.