Given:
Given that the first term of the geometric sequence is 729.
The common ratio is

We need to determine the seventh term of the sequence.
Seventh term:
The seventh term of the sequence can be determined using the formula,

To find the seventh term, let us substitute n = 7 in the above formula, we get;
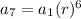
Now, substituting
 and
and
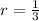 , we get;
, we get;
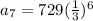
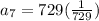

Thus, the seventh term of the geometric sequence is 1.