Question

Calculate the area of the shaded region. Use 3.14 for pi. Round your answer to the nearest hundredth if necessary

Answer 1

2.5m--->250cm
Diameter of circle =>250cm

Radius of circle =>125 cm

Area of circle=>3.14 x (125x125) = 49062.5cm

49062.5cm equals to 490.625m

Area of square=> 2.5 x2.5 = 6.25

490.625-6.25= 484.375m

Rounded to nearest hundreth,
484.375m~484.38m

Final.answer : 484.38m squared

Answer 2

Based on the diagram shown above, the area of the shaded region rounded to the nearest hundredth is 1.34 square meter.

In Mathematics and Euclidean Geometry, the area of a square can be calculated by using this formula;

A =
`s^2`

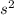
Where:

• A is the area of a square.
• s is the side length of a square.

By substituting the given side lengths into the formula, we have;

Area of square, A = 2.5 × 2.5

Area of square, A = 6.25 square meters.

In Mathematics and Geometry, the area of a circle can be calculated by using this formula:

Area of circle = π
`r^2`

Where:

r represents the radius of a circle.

By substituting, we have;

Radius of circle = diameter/2 = 2.5/2

Radius of circle = 1.25 m.

Area of circle = π ×
`1.25^2`

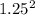
Area of circle ≈ 4.91 square meters.

Area of the shaded region = Area of square - Area of circle

Area of the shaded region = 6.25 - 4.91

Area of the shaded region = 1.34 square meter.