Question

Write the point-slope form of the equation of the line through the points (1,-1) and (5,-2)

Answer 1

y + 1 = -1/4 (x - 1) Answer choice C is correct.

Explanation:

Point Slope Formula: y - y1 = m (x-x1)

Your points:

(1,-1) and (5,-2)

You need to find the slope first:

Use the formula: y2 - y1 / x2 - x1

Your y2 is -2

y1 is -1

x2 is 5

x1 is 1

-2 - (-1)/5-1

-2 + 1 /4

-1/4 is your slope and the "m" in the formula.

Now we know our y1 is -1 and x1 is 1 you just need to plug them in

y + 1 = -1/4 (x - 1)

Notice that I didn't write y - (-1) this is because the negatives cancel into positives.

Answer choice C is correct.

Answer 2

c.
`y+1=-(1)/(4) (x-1)`

Explanation:

Hi there!

We are given the points (1, -1) and (5, -2)

We want to find the equation of that line using those points, in point-slope form

Point-slope form is written as
`y-y_1=m(x-x_1)`, where m is the slope and
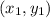`(x_1, y_1)` is a point

First, let's find the slope of the line

The formula for the slope (m) calculated from 2 points is
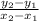`(y_2-y_1)/(x_2-x_1)`, where
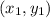`(x_1, y_1)` and
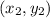`(x_2, y_2)` are points

We already have everything we need to find the slope, but let's label the values of the points to avoid any confusion when calculating.

`x_1=1\\y_1=-1\\x_2=5\\y_2=-2`

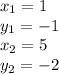
Now substitute:

m=
`(y_2-y_1)/(x_2-x_1)`

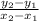
m=
`(-2--1)/(5-1)`

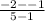
Subtract

m=
`(-2+1)/(5-1)`

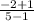
m=
`(-1)/(4)`

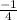
The slope of the line is -1/4

Now substitute this into the formula to find point-slope form (remember that this is
`y-y_1=m(x-x_1)`, and that m is the slope value)

Therefore:

`y-y_1=-(1)/(4) (x-x_1)`

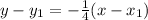
Now, let's substitute the values of
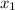`x_1` and
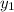`y_1`, which we found earlier (which are 1 and -1 respectively) into the equation

`y--1=-(1)/(4) (x-1)`

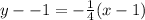
Simplify

`y+1=-(1)/(4) (x-1)`

This equation matches option c, which is the answer.
Hope this helps!