Question

What are the coordinates of the point on the directed line segment from (3, -1)(3,−1) to (9, 8)(9,8) that partitions the segment into a ratio of 1 to 2?

Answer 1

Answer

Explanation:

(5,2)

Answer 2

Coordinates of the point on the directed line segment is (15/3,7/3)

Explanation:

Given:

A line segment with coordinates partition in some ratio.

Lets say that the ends of the segment are A and B.

We have to find the coordinates of C.

Coordinates of point A are = (3,-1)

Coordinates of point B are = (9,8)

Considering the point C as (x,y) and the partition ratios as m:n i.e 1:2

Formula to be used:

Section formula:

• 
  `x= (mx_2+n_x_1)/(m+n),\ y=(my_2+ny_1)/(m+n)`

Using the above formula.

⇒
`x= (mx_2+n_x_1)/(m+n),\ y=(my_2+ny_1)/(m+n)`

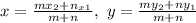
⇒ Plugging the values.

⇒
`x= (1(9)+2(3))/(1+2),\ y=(1(8)+2(-1))/(1+2)`

⇒
`x= (9+6)/(3),\ y=(9-2)/(3)`

⇒
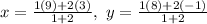
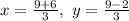
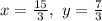`x= (15)/(3),\ y=(7)/(3)` or

⇒
`x= 5,\ y=2.3`

The coordinates of the point on the directed line segment is (15/3,7/3) in terms of decimals it is (5,2.3).