Answer: 832 inches squared.
Explanation:
Composite shapes' surface areas can be found by taking the individual geometrical shapes' surface areas. After doing so, add together the surface area, but make sure to subtract any overlapping area.
For this instance, you have a cube placed on top of a rectangular prism.
A cube's surface area is defined as
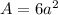 ., in which
., in which
 is defined as the side length of the cube.
is defined as the side length of the cube.
A rectangular prism's surface area is defined as
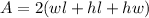 , in which the side lengths of the width, length, and height are used as W, L, and H.
, in which the side lengths of the width, length, and height are used as W, L, and H.
The cube's surface area when the side length is 9 inches is 6 * (9)^2, or 486 inches squared.
The rectangular prism's surface area when side lengths are 14, 11, and 4 inches is A = 2(11*14 + 4*14 + 11*4), or 508 inches squared.
To calculate the composite area, the areas of the overlapping sections must be subtracted. Because there is a 9x9 area on the cube and a 9x9 area on the prism overlapping, you must subtract 2* (9)^2, or 162 inches squared, from the total area of 994 inches.
994 - 162 = 832 inches squared.