Answer:
1. x = +/- 2
 - 7
- 7
2. x =
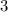 +/-
+/-
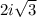
3. n =
 +/-
+/-
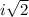
Explanation:
1. Divide both sides by 2: (x + 7)^2 = 8
Square root both sides: x + 7 = +/- 2

Subtract 7 from both sides: x = +/- 2
 - 7
- 7
2. Square root both sides: x - 3 =
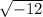
Since there is a negative inside the radical, we need to have an imaginary number:
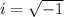 . So,
. So,
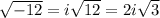
Add 3 to both sides: x =
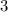 +/-
+/-
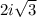
3. Divide by -5 from both sides: (n - 2)^2 = -2
Square root both sides: n - 2 =
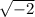
Again, we have to use i:
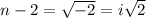
Add 2 to both sides: n =
 +/-
+/-
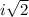
Hope this helps!