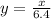Answer:
Explanation:
The complete question in the attached figure
we know that
A relationship between two variables, x, and y, represent a proportional variation if it can be expressed in the form
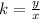 or
or
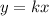
In this problem, we have a proportional relationship between the average speed (x) and the time (y)
Find the constant of proportionality k
Fox =32, y=5
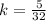
substitute
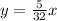
we have
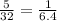
therefore