Question

Use the quadratic formula
to solve equation
x²-2x=-9

Answer 1

Answer: " x = 1 ± 2i√2 " .

_________________________________

Explanation:

_________________________________

To solve the given equation:

"
`x^(2) -2x = -9` " ;

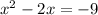for all values of "
`x`" ; by using the "quadratic formula equation" ;

we must first rewrite the given equation in "quadratic format" ;

→ that is; in the format:

→ "
`ax^(2) +bx + c= 0` " ; {
`a\\eq 0}`} ;

________________________________

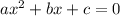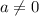So, given:

"
`x^(2) -2x = -9` " ;

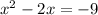→ We must add "9" to Each Side of the equation; to get the equation into "quadratic format" ; as follows:

→ x² − 2x + 9 = -9 + 9 ;

to get:

→ " x² − 2x + 9 = 0 " ;

___________________________________

→ which is written in the "quadratic format" ; that is:

→ "
`ax^(2) +bx + c= 0` " ; {
`a\\eq 0`} ;

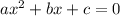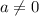in which:

"a = 1" ; [the "implied coefficient; "1" ; since "1" multiplied by "any value" ;

results in "that same value" ;

→ {Note this this is known as the "identity property"

of multiplication.}.

b = -2 ;

c = 9 ;

and since: " a = 1 " ; We know that: " {a≠0.}. "

_______________________________________

To solve for all value(s) for "
`x`" in our given equation by using the quadratic equation formula:

→ We use the following quadratic equation formula:

________________________________________

→ x = [-b ± √(b² − 4ac) ] / [2a] ;

Note that in the "quadratic formula" :

→ "
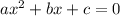`ax^(2) +bx + c= 0` " ; "a ≠ 0" ;

→ Since we divide by "2a" {refer to the "denominator"];

→ and if: "a" were to equal "0" ; then the "denominator" would equal: "2a = 2*a = 2*0 = 0" ; and we cannot divide by "0" .

______________________________________________

So, let us solve for the values for "
`x`" ; by plugging in our known values into the quadratic equation formula:

______________________________________________

→
`x` = [
`-b` ±
`\sqrt{(b^(2)-4ac)} ] / [2a] ;`

So, to solve for "x" ; plug in "1" for "a"; "-2" for "b" ; "9" for "c" ;

→ So: "
`(-b) = -(-2) = 2` " ;

" b² = (-2)² = (-2) * (-2) = 4 " ;

" 4ac = 4 * 1 * 9 = 4 * 9 = 36 ;

" 2a = 2 * 1 = 2 " ;

__________________________________________

So:

→ x = (2 ± √(4−36) / 2 ;

Note: 4−36 = -32 ;

So:

→ x = (2 ± √-32) / 2 ;

Note: √-32 ; can be written as " i * √-32" ; or simply: "i√-32" ; since "i" is an 'imaginary number" that can represent the imaginary number: "√-1" ;

→ since: "√-32 = √-1 * √32" ; if: "√-1" really existed.

__________________________________________

→ Now, we can further simplify:

→ i√32;

_________________________________________

Let us simplify: "√32" ;

→ √32 = √16 * √2 = 4 * √2 ; or, write as 4√2 ;

So: √-32 = i√-32 = i * 4√2 = 4i√2 .

_________________________________________

We have, from above:

→ x = (2 ± √-32) / 2 ;

Rewrite—by substituting "4i√2" ; in lieu of: "√-32" ; as following

→ x = (2 ± 4i√2) / 2 ;

We are dividing the numerator by "2" ; so we can further simplify:

to get:

________________________________________

→ " x = 1 ± 2i√2 " .

________________________________________

Hope this answer—and explanation—is helpful!

Wishing you well in your academic endeavors!

________________________________________