Question

. A random sample of 80 jars of grape jelly has a mean weight of 568 g, with a standard deviation of 48.28 g. Construct a 99% confidence interval for , the true weight of a jar of jelly.

Answer 1

The 99% confidence interval is (553.7523, 582.2477)

Explanation:

The formula for the confidence interval is

`CI=\bar{x}\pm z(s)/(√(n))`

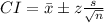
Where:

s = Standard deviation

z = t
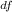`_(df)` since the population variance is unknown

df = Degrees of freedom

n = Sample count

Which gives

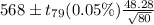
`568 \pm t_(79)(0.05\%) (48.28)/(√(80) )`

= 568
`\pm` 2.64×5.4

(553.7523, 582.2477)

That is there is a 99% chance that the mean weight of a jar of jelly is

553.75 < μ < 582.25.