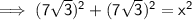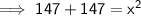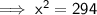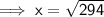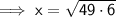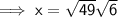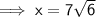Question

Find x on the triangle geometry please help

Answer 1

`\\ \rm\Rrightarrow cos\theta=(Base)/(Hypotenuse)`

`\\ \rm\Rrightarrow cos45=(7√(3))/(x)`

`\\ \rm\Rrightarrow (1)/(√(2))=(7√(3))/(x)`

`\\ \rm\Rrightarrow x=7√(3)√(2)`

`\\ \rm\Rrightarrow x=7√(6)`

Answer 2

`\sf x=7√(6)`

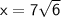
Explanation:

As the base angles of the triangle are the same, then the legs of the right triangle are also equal. Therefore, we can use Pythagoras' Theorem to calculate x.

Pythagoras' Theorem: a² + b² = c²

(where a and b are the legs, and c is the hypotenuse of the right triangle)

Given:

• a = 7√3
• b = 7√3
• c = x

Substituting the given values into the formula:

`\sf \implies (7√(3))^2+(7√(3))^2=x^2`

`\sf \implies147+147=x^2`

`\sf \implies x^2=294`

`\sf \implies x=√(294)`

`\sf \implies x=√(49 \cdot 6)`

`\sf \implies x=√(49)√(6)`

`\sf \implies x=7√(6)`