Answer:
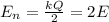
If the charge is doubled, the electric field is also doubled.
Step-by-step explanation:
Electric field due to the negative charge is given as:
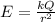
where k = Coulomb's constant
Q = electric charge
r = distance between charge and point of consideration
At 2 m from the negative charge, the magnitude of the Electric field due to a negative charge -Q is given as E:
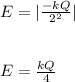
If the charge is doubled, the new charge becomes -2Q and the new electric field becomes:
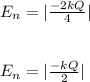
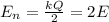
If the charge is doubled, the electric field is also doubled.