Question

Jacobs college savings are invested in a bond that pays an annual interest of 6.2% compounded continuously. How long will it take for the money to triple

Answer 1

Therefore the value of bond will triple after 17.72 years.

Explanation:

The formula of Compounded continuously

`A=Pe^(rt)`

A= Amount after t year

P= initial amount

r = rate of interest

t= time in year.

Given that,

Jacobs college saving are invested in bond that pay 6.2% compounded continuously.

Let after t years the initial amount P will be triple i.e 3P.

Here P=P, A=3P, r= 6.2%=0.062

`\therefore 3P=Pe^(0.062t)`

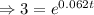`\Rightarrow 3=e^(0.062t)` [ Multiply
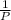`\frac 1P` both sides]

Taking ln both sides

`\Rightarrow ln3=ln(e^(0.062t))`

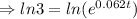
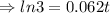`\Rightarrow ln3={0.062t}` [ since
`ln(e^a)=a` ]

`\Rightarrow t=(ln3)/(0.062)`

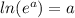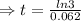
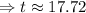`\Rightarrow t\approx 17.72` years

Therefore the value of bond will triple after 17.72 years.