Question

For each of the following functions, determine if they are injective. Also determine if theyare surjective. Also determine if they are bijective.No justification needed.The firstthree have already been filled out for you, as an example. Also, recall interval notation:[a,b] ={x∈R:a≤xandx≤b}. You should be able to come up with a similar definitionfor (a,b) and (a,b] and [a,b).1.f:R→R, f(x) =x2.f:R≥0→R, f(x) =x+ 13.f:R≥0→R, f(x) = cos(x)4.f:R→R, f(x) =ex5.f:R→R, f(x) =2xifx≥0−x2ifx <0.6.f:R→(0,[infinity]), f(x) =ex7.f:R≥0→R≥0, f(x) =x48.f:{−1,2,−3}→{1,4,9}, f(x) =x29.f:R≥0→[−1,1], f(x) = cos(x)10.f: [0,π]→[−1,1], f(x) = cos(x)11.f:R≥0→[−1,1], f(x) = 012.f: US Citizens→Z, f(x) = the SSN ofx.13.f: US Zip Codes→US States, f(x) = The state thatxbelongs to.

Answer 1

Check below

Explanation:

1. Definition for intervals

`(a,b)=\left \{ x\in\Re : a<x \:and \:x<b \right \}\\(a,b]=\left \{ x\in\Re : a<x \:and \:x\leq b \right \}`

2. Functions

1)
`\Re \rightarrow \Re \\ f(x)=x`

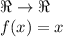
Let's perform graph tests.

That's an one to one, injective function. Look how any horizontal line touches that only once. Also, It's a surjective and a bijective one.

2)
`\Re\geq0\rightarrow\Re , f(x)=x+1\\`

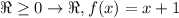
Injective, surjective and bijective.

Injective: a horizontal line crosses the graph in one point.

3)
`f:\Re\geq 0\rightarrow\Re, f(x) = cos(x)`

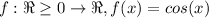
The cosine function is not injective, bijective nor surjective.

4)
`f:\Re\rightarrow\Re \:f(x)=ex`

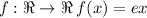
Since e, is euler number it's a constant. It's also injective, surjective and bijective.

5) Quite unclear format

`6) \:f:\Re\rightarrow(0,\infty), f(x) =ex`

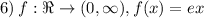
Despite the Restriction for the CoDomain, the function remains injective, surjective and therefore bijective.

`7) f:\Re\geq 0 \rightarrow \Re\geq0, f(x) =x^(4)`

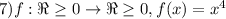
Not injective nor surjective therefore not bijective too.

`8).f:\{-1,2,-3\}}\rightarrow \{1,4,9\}, f(x) =x^(2)`

`f(-1)=1, f(2)=4, f(-3)=9`

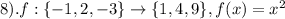
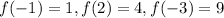
Injective (one to one), Surjective, and Bijective.

`10) f:\Re\geq 0\rightarrow [-1,1], f(x)= cos(x)\\-1=cos(x) \therefore x=\pi,3\pi,5\pi,etc.`

Surjective.

`11.f:R\geq 0[-1,1], f(x) = 0\\`

Surjective

12.f: US Citizens→Z, f(x) = the SSN of x.

General function

13.f: US Zip Codes→US States, f(x) = The state that x belongs to.

Surjective