Answer:
Explanation:
Hello!
The mean life of 16 lithium batteries was estimated with a 95% CI:
(628.5, 661.5) hours
Assuming that the variable "X: Duration time (life) of a lithium battery(hours)" has a normal distribution and the statistic used to estimate the population mean was s Student's t, the formula for the interval is:
[X[bar]±
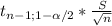 ]
]
The amplitude of the interval is calculated as:
a= Upper bond - Lower bond
a= [X[bar]+
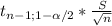 ] -[X[bar]-
] -[X[bar]-
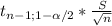 ]
]
and the semiamplitude (d) is half the amplitude
d=(Upper bond - Lower bond)/2
d=([X[bar]+
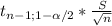 ] -[X[bar]-
] -[X[bar]-
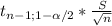 ] )/2
] )/2
d=
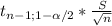
The sample mean marks where the center of the calculated interval will be. The terms of the formula that affect the width or amplitude of the interval is the value of the statistic, the sample standard deviation and the sample size.
Using the semiamplitude of the interval I'll analyze each one of the posibilities to see wich one will result in an increase of its amplitude.
Original interval:
Amplitude: a= 661.5 - 628.5= 33
semiamplitude d=a/2= 33/2= 16.5
1) Having a sample with a larger standard deviation.
The standard deviation has a direct relationship with the semiamplitude of the interval, if you increase the standard deviation, it will increase the semiamplitude of the CI
↑d=
 * ↑S/√n
* ↑S/√n
2) Using a 99% confidence level instead of 95%.
d=
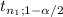 * S/√n
* S/√n
Increasing the confidence level increases the value of t you will use for the interval and therefore increases the semiamplitude:
95% ⇒
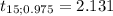
99% ⇒
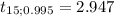
The confidence level and the semiamplitude have a direct relationship:
↑d= ↑
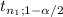 * S/√n
* S/√n
3) Removing an outlier from the data.
Removing one outlier has two different effects:
1) the sample size is reduced in one (from 16 batteries to 15 batteries)
2) especially if the outlier is far away from the rest of the sample, the standard deviation will decrease when you take it out.
In this particular case, the modification of the standard deviation will have a higher impact in the semiamplitude of the interval than the modification of the sample size (just one unit change is negligible)
↓d=
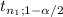 * ↓S/√n
* ↓S/√n
Since the standard deviation and the semiamplitude have a direct relationship, decreasing S will cause d to decrease.
4) Using a 90% confidence level instead of 95%.
↓d= ↓
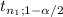 * S/√n
* S/√n
Using a lower confidence level will decrease the value of t used to calculate the interval and thus decrease the semiamplitude.
5) Testing 10 batteries instead of 16. and 6) Testing 24 batteries instead of 16.
The sample size has an indirect relationship with the semiamplitude if the interval, meaning that if you increase n, the semiamplitude will decrease but if you decrease n then the semiamplitude will increase:
From 16 batteries to 10 batteries: ↑d=
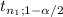 * S/√↓n
* S/√↓n
From 16 batteries to 24 batteries: ↓d=
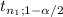 * S/√↑n
* S/√↑n
I hope this helps!