Question

Find AC
What Is The Equation To AC?

Answer 1

Given:

Given that ABC is a right triangle.

The length of AB is 7 units.

The measure of ∠A is 65°

We need to determine the length of AC

Length of AC:

The length of AC can be determined using the trigonometric ratio.

Thus, we have;

`cos \ \theta=(adj)/(hyp)`

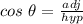
Where the value of
`\theta` is 65° and the side adjacent to the angle is AC and the side hypotenuse to the angle is AB.

Substituting the values, we have;

`cos \ 65^(\circ)=(AC)/(AB)`

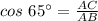
Substituting AB = 7, we have;

`cos \ 65^(\circ)=(AC)/(7)`

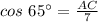
Multiplying both sides by 7, we get;

`cos \ 65^(\circ) * 7=AC`

`0.423 * 7=AC`

`2.961=AC`

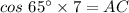
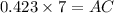
Rounding off to the nearest hundredth, we get;

`2.96=AC`

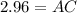
Thus, the length of AC is 2.96 units.