1. Slope :
![\[ m = 1.4 \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/fcurnqtkuyk0mcq6imb56b1prev596ltmb.png)
2. The equation:
![\[ y = 1.4x + 7 \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/nj41ssh35vymccgg4jbuj7an09x6l4mdm8.png)
To write the equation of the line that passes through the points
 and
and
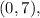 we will follow these steps:
we will follow these steps:
1. Calculate the slope (m) of the line using the formula:
![\[ m = (y_2 - y_1)/(x_2 - x_1) \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/ga9scnub28k1t3ld1a45yfpcctcex36osp.png)
where
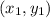 and
and
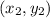 are the coordinates of the two points.
are the coordinates of the two points.
2. Substitute the coordinates of the points into the formula to find the slope.
3. Use the slope and one of the points to write the equation of the line in point-slope form:
![\[ y - y_1 = m(x - x_1) \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/q63fbhqii6pllg76ls82xufftnsy24ascb.png)
Then solve for y to get the slope-intercept form:
![\[ y = mx + b \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/wtsqddmv4ciat8euuj6md8p7n5le92wt38.png)
where
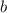 is the y-intercept.
is the y-intercept.
4. Since we have the point
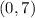 , which is where the line crosses the y-axis, the y-intercept
, which is where the line crosses the y-axis, the y-intercept
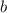 is 7.
is 7.
Let's carry out these calculations.
The slope (m) of the line is 1.4, and the y-intercept (b) is 7. Therefore, the equation of the line in slope-intercept form is:
![\[ y = 1.4x + 7 \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/nj41ssh35vymccgg4jbuj7an09x6l4mdm8.png)
Here are the step-by-step calculations:
1. Slope calculation:
![\[ m = (7 - 0)/(0 - (-5)) = (7)/(5) = 1.4 \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/9pchqhbbvsbyomlpsrxx97a8dm3ypwfsgk.png)
2. Using the slope and the y-intercept, the equation becomes:
![\[ y = 1.4x + 7 \]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/nj41ssh35vymccgg4jbuj7an09x6l4mdm8.png)