Answer:
$18,726.11
Explanation:
Lets use the compound interest formula provided to solve this:
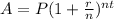
P = initial balance
r = interest rate (decimal)
n = number of times compounded annually
t = time
First lets change 9% into a decimal:
9% ->
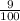 -> 0.09
-> 0.09
Since the interest is compounded quarterly, we will use 4 for n. Lets plug in the values now:

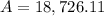
The balance after 5 years is $18,726.11