Question

The Pew Research Center interviewed a random sample of 1,546 adult Americans to determine the average time they spent sleeping per night. The sample mean was found to be 7.2 hours of sleep, with a sample standard deviation of 1.4 hours. Construct a 95% confidence interval for the true mean. Interpret your confidence interval in words.

Answer 1

CI=[ 7.1302,7.2698]

we are 95% confident that the true mean sleeping time lies between the interval[ 7.1302,7.2698]

Explanation:

-Given that the sample size, n=1546 the mean is 7.2 hrs and the standard deviation,
`\sigma=1.4`

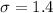
-The 95% confidence interval can be calculated using the formula:

`CI=\mu\pm z_(\alpha/2)(\sigma)/(√(n))\\\\=\mu\pm z_(0.025)* (\sigma)/(√(n))\\\\=7.2\pm1.96* (1.4)/(√(1546))\\\\=7.2\pm 0.0698\\\\CI=[7.1302, \ 7.2698]`

The confidence intervals is therefore 7.1302,7.2698]

Hence, we are 95% confident that the true mean sleeping time lies between the interval[ 7.1302,7.2698]