Question

I need help with part b. I feel like there’s a catch, I want to do the first derivative test, however, I feel like there is a better way.

Answer 1

The fifth degree Taylor polynomial of g(x) is increasing around x=-1

Explanation:

Yes, you can do the derivative of the fifth degree Taylor polynomial, but notice that its derivative evaluated at x =-1 will give zero for all its terms except for the one of first order, so the calculation becomes simple:

`P_5(x)=g(-1)+g'(-1)\,(x+1)+g`

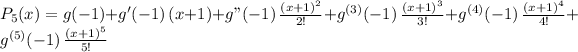
and when you do its derivative:

1) the constant term renders zero,

2) the following term (term of order 1, the linear term) renders:
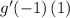`g'(-1)\,(1)` since the derivative of (x+1) is one,

3) all other terms will keep at least one factor (x+1) in their derivative, and this evaluated at x = -1 will render zero

Therefore, the only term that would give you something different from zero once evaluated at x = -1 is the derivative of that linear term. and that only non-zero term is:
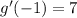`g'(-1)= 7` as per the information given. Therefore, the function has derivative larger than zero, then it is increasing in the vicinity of x = -1