3,628,800 ways can they arrange themselves in the shuttle, If 10 friends are going to occupy 10 seats in shuttle on the way to the airport.
Step-by-step explanation:
The given is,
10 friends are going to occupy 10 seats in shuttle on the way to the airport
Step:1
No. of friends - 10
No.of seats in space shuttle - 10
Formula to calculate the no. of ways to arrange,
 ............................(1)
............................(1)
Where, n - Positive integer
Step:2
From given, n = 10
Equation (1) becomes,
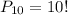
= 10 × 9 × 8 × 7 × 6 × 5 × 4 × 3 × 2 × 1
= 3628800
= 3628800 ways
Result:
3,628,800 ways can they arrange themselves in the shuttle, If 10 friends are going to occupy 10 seats in shuttle on the way to the airport.