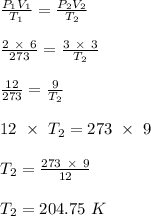Question

A sample of gas is at 273°K and 2.00 atm with a volume of 6.00 liters. When the gas is compressed to 3.00 liters and the pressure increases to 3.00 atm, what is the new temperature of the sample?

Answer 1

The new temperature of the sample of gas, given that the pressure increases to 3.00 atm is 204.75 K

How to calculate the new temperature of the gas?

A good look a the question given above, shows that the new temperature of the sample of gas can be calculated using the combined gas equation. This is shown below:

• Initial temperature of gas sample (T₁) = 2732 K
• Initial pressure of gas sample (P₁) = 2.00 atm
• Initial volume of gas sample (V₁) = 6.00 liters
• New volume of gas sample (V₂) = 3.00 liters
• New pressure of gas sample (P₂) = 3.00 atm
• New temperature of gas sample(T₂) = ?

`(P_1V_1)/(T_1) = (P_2V_2)/(T_2) \\\\(2\ *\ 6)/(273) = (3\ *\ 3)/(T_2)\\\\(12)/(273) = (9)/(T_2)\\\\12\ *\ T_2 = 273\ *\ 9\\\\T_2 = (273\ *\ 9)/(12) \\\\T_2 = 204.75\ K`

Answer 2

Answer: 0.00488K

Explanation: using the general gas equation

(P1V1)/T1 =(P2V2)/T1

Substitute

2*6/273= 3*3/T

Simplify

T= 0.00488°K