Question

= The average score of Karl's first four math tests is 72 points. After the fifth and the sixth tests, his average score increased to 75 points. If the score of his sixth test is 4 points more than the score of his fifth test, how many points did he score on his sixth test?

Answer 1

83 points

Explanation:

-The formula for average is given as:

`\bar X=(1)/(n)\sum{x_i}\\\\x_i=Individual \ Test \ scores\\n=number \ of \ scores`

Let a be his 5th score and (a+4) of the 6th

#We subtract the sum of the first 4 tests from the 6, to get the sum of the 5th and 6th tests:

`(6^(th)+5^(th))=\bar X n_6-\bar X n_4\\\\=75* 6-72* 4\\\\=162`

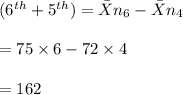
#We substitute for the 5th and 6th to solve a:

`5^(th)=a\\6^(th)=a+4\\\\a+(a+4)=162\\\\2a=158\\\\a=79\\\\6^(th)=a+4=79+4=83`

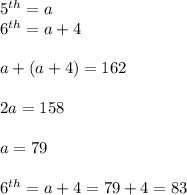
Hence, Karl score 83 points in his 6th test.