Green hose takes 3 hours to fill her hot tub and Red hose takes 6 hours to fill her hot tub.
Explanation:
The given is,
Red hose takes 3 hours more than if she only use green hose
She uses both hoses together, the hot tube fills in 2 hours
Step:1
Let, x - Hours taken by green hose to fill her tub
From given,
Time taken by red hose = (x + 3) Hours
Time taken by both hoses = 2 hours
One hour work,
One hour work of green hose =
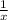
One hour work of Red hose =
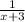
One hour work both hoses uses together =

One hour work if she use both hoses together
= One hour work of green hose + One hour work of red hose
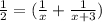
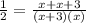
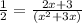

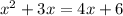
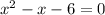
Solving the above equation,
x = 3
From the x value,
Hours taken by green hose to fill her tub, x = 3 hours
Time taken by red hose = (x + 3) Hours
= 3 + 3 = 6 hours
Hours taken by Red hose to fill her tub = 6 hours
Result:
Green hose takes 3 hours to fill her hot tub and Red hose takes 6 hours to fill her hot tub.