Question

A rock is in the shape of a sphere with a radius of 5.08 centimeters. The rock has a density of 5.5 grams per cubic centimeter. Find the mass of the rock to the nearest whole gram.

Answer 1

The mass of the rock is 3020.27 grams , if a rock is in the shape of a sphere with a radius of 5.08 centimeters and the rock has a density of 5.5 grams per cubic centimeter,

Explanation:

The given is,

Radius of the rock is 5.08 cm (Sphere)

Density of rock 5.5 grams per cubic centimeters

Step:1

Formula for volume of sphere,

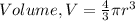`Volume, V= (4)/(3) \pi r^(3)`..........................(1)

Where, r - Radius of sphere

From the given values,

r = 5.08 cm

Equation (1) becomes,

`V= (4)/(3) \pi (5.08)^(3)`

`V= (4)/(3) (3.1415) (5.08)^(3)`

`= (4)/(3) (3.1415) (131.097)`

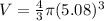
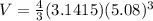
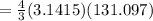
V = 549.14 cubic centimeters

Step:2

Mass of rock = Volume of rock × Density of rock

= 549.14 × 5.5 ( ∵
`cm^(3) (( gram)/(cm^(3)) ) = gram` )

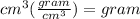= 3020.27 grams

Mass of the rock = 3020.27 grams

Result:

The mass of the rock is 3020.27 grams , if a rock is in the shape of a sphere with a radius of 5.08 centimeters and the rock has a density of 5.5 grams per cubic centimeter,