To solve this, you need to isolate/get the variable "x" by itself in the inequality:
3x + 2.4 ≥ 3.0 First subtract 2.4 on both sides
3x + 2.4 - 2.4 ≥ 3.0 - 2.4
3x ≥ 0.6 Now divide 3 on both sides to get "x" by itself
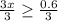
x ≥ 0.2 Your answer is the 1st option