Question

A fossil was analyzed and determined to have a carbon-14 level that is 80 % that of living organisms. the half-life of c-14 is 5730 years. how old is the fossil? express your answer with the appropriate units.

Answer 1

The fossil is 1860 years old.

Explanation:

The equation for the amount of fossil has the following format:

`Q(t) = Q(0)e^(rt)`

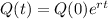
In which Q(t) is the amount after t years, Q(0) is the initial amount and r is the rate of change.

Half-life of c-14 is 5730 years.

This means that
`Q(5730) = 0.5Q(0)`

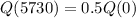
So

`Q(t) = Q(0)e^(rt)`

`0.5Q(0) = Q(0)e^(5730r)`

`e^(5730r) = 0.5`

`\ln{e^(5730r)} = ln(0.5)`

`5730r = ln(0.5)`

`r = (ln(0.5))/(5730)`

`r = -0.00012`

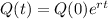
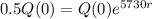
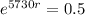
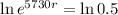
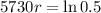
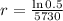
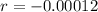
So

`Q(t) = Q(0)e^(-0.00012t)`

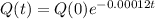
How old is the fossil?

This is t for which

`Q(t) = 0.8Q(0)`

So

`Q(t) = Q(0)e^(-0.00012t)`

`0.8Q(0) = Q(0)e^(-0.00012t)`

`e^(-0.00012t) = 0.8`

`\ln{e^(-0.00012t)} = ln(0.8)`

`-0.00012t = ln(0.8)`

`t = -(ln(0.8))/(-0.00012)`

`t = 1860`

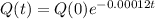
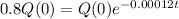
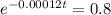
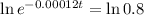
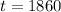
The fossil is 1860 years old.