Question

Pudge has three times as many apples as Ace, and Ace has twice as many as Christi. How many apples does each have if pudge has 12 more than both Ace and Christi together?

Answer 1

Pudge has 12 morethan apples that is 24 apples and both Ace and Christi has not more than 12 apples together they has atmost 12 apples.The apples does each having is

P = Pudge's Apples =24

A = Ace's Apples =8

and C = Christi's Apples=4.

Explanation:

Let P = Pudge's Apples

Let A = Ace's Apples

Let C = Christi's Apples

To find how many apples does each have if Pudge has 12 more than both Ace and Christi together:

Given that P = 3A , A = 2C and P = A + C + 12

Substitute the value for P in P = A + C + 12 we get

3A = A + C + 12

3A-A=C+12

2A=C+12

From A = 2C we have that
`C=(A)/(2)`

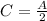
Substitute the value C:

`2A=(A)/(2)+12`

`2A-(A)/(2)=12`

`(4A-A)/(2)=12`

`(3A)/(2)=12`

`3A=12* 2`

`A=(24)/(3)`

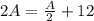
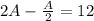
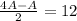
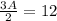
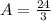
∴ A=8

Substituting the value of A in P=3A we get

P = 3(8)

∴ P = 24

Substituting the values of P and A in P = A + C + 12

`24=8+C+12`

`24=C+20`

`24-20=C`

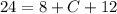
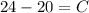
4=C

Rewritting we get

∴ C=4

Hence Pudge has 12 morethan apples that is 24 apples

and both Ace and Christi has not more than 12 apples together they has atmost 12 apples