Question

Retirement savings

A couple thinking about retirement decides to put aside $3,000 each year in a savings plan that earns 8% interest. In 5 years, they will receive a gift of $10,000 that also can be invested.

a. How much money will they have accumulated 30 years from now?

b. If the goal is to retire with $800,000 savings, how much extra do they need to save every year?

Answer 1

a. $408,334.39

b. $3,457.40

Step-by-step explanation:

r = rate per period = 8% = 0.08

P = Initial Value of Gift = $10,000

t = time = 30 - 5 = 25, As received after 5 years.

`A = P (1 + r)^(t)`

`A = $10,000 (1 + 0.08)^(25)`

`A = $10,000 x 1.08^(25)`

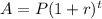
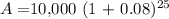
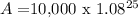
A = $10,000 x 6.8485

A = $68,484.75

`FV of annuity = P [((1 + r)^(n) - 1)/(r) ]`

P = Periodic Payment = $3,000

a.

n = number of periods = 30

`FV of annuity = 3,000 [((1 + 0.08)^(30) - 1)/(0.08) ]`

`FV of annuity = 3,000 [((1.08)^(30) - 1)/(0.08) ]`

`FV of annuity = 3,000 [\frac{10.0627 - 1} {0.08} ]`

`FV of annuity = 3,000 [\frac{9.0627} {0.08} ]`

FV of annuity = $3,000 x 113.2832

FV of annuity = $339,849.63

Accumulated value of money can be calculated as follows;

$68,484.75 + $339,849.63

$408,334.39

b.

If they wish to retire with $800,000 savings, they need to save additional amount of money every year to provide additional amount of money, as follows;

$800,000 - $68,484.75

$731,515.24

The extra annual savings can be calculated as follows;

`731,515.24 = P [((1 + 0.08)^(30) - 1 )/(0.08) ]`

$731,515.24 = P x 113.28

Divide the above equation by 113.28 we get;

`P = (731,515.24)/(113.28)`

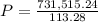
P = $6,457.40

They are already paying $3,000, So the extra saving they need make every year is calculated as follows;

$6,457.40 - $3,000

$3,457.40