Question

A couple plans to have three children. Each child is equally likely to be a girl or​ boy, with gender independent of that of the other children. a. Construct a sample space for the genders of the​ children, using B for boy and G for girl. b. Find the probability that all 3 of the children are boys. c. Answer part b​ if, in​ reality, for a given​ child, the chance of a boy is 0.51.

Answer 1

a) X={BBB;BBG:BGG:GGG}

b) P(BBB)=0.125

c) P(BBB)=0.133

Explanation:

The sample space states all the possible values that the random variable can take. In this case, the order does not matter, so the possible combinations for the random variable are:

X={BBB;BBG:BGG:GGG}

The probability p of having a boy is p=0.5, as it is equally likely to have a girl or a boy.

The probability that all 3 children are boys can be calculated multiplying 3 times the probability of having a boy. That is:

`P(X=BBB)=p\cdot p\cdot \cdot p =p^3=0.5^3=0.125`

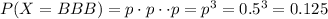
In the case that the chance of having a boy is p'=0.51, the probabiltity of having 3 boys become:

`P(X=BBB)=p'^3=0.51^3=0.133`